Physics Lesson 15.1.4 - Appendix - Charge Density, Electric Field and Gauss Law

Please provide a rating, it takes seconds and helps us to keep this resource free for all to use

Welcome to our Physics lesson on Appendix - Charge Density, Electric Field and Gauss Law, this is the fourth lesson of our suite of physics lessons covering the topic of Electric Current. Current Density, you can find links to the other lessons within this tutorial and access additional physics learning resources below this lesson.

Appendix - Charge Density, Electric Field and Gauss Law

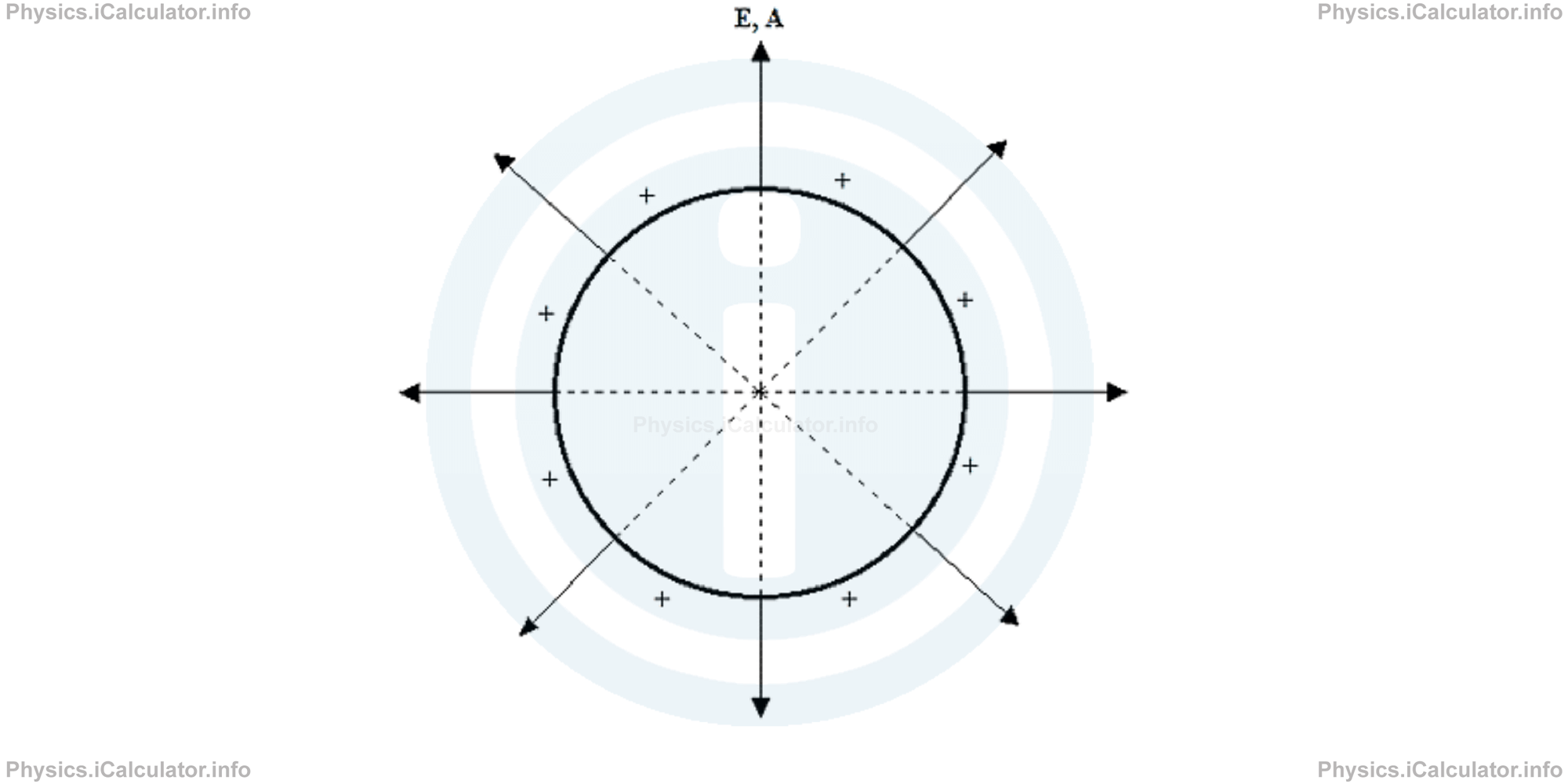
Now that we explained the meaning of charge density in all possible forms (linear, surface and volume charge density), let's recall Gauss Law and extend its explanation further by taking into consideration the new concepts. Remember that Gauss Law involves electric flux Φ and its general mathematical form is

while the formula derived directly from definition of electric flux is

where E is the electric field, A is the area vector and θ is the angle between the area vector and electric field vector.

a. Transforming Gauss Law using the surface charge density concept

Let's consider a charged conductor of surface area A. Its surface charge density obviously is σ = Q/A where Q is the charge. From Gauss Law, we know that

For simplicity, let's consider the field lines as parallel to the area vector, i.e. cos θ = 1 because θ = 0⁰.

Given that Q = σ ∙ A, we can write

Simplifying the area A from both sides, we obtain for the electric field in terms of surface charge density

The above formula means that the magnitude of electric field outside a conductor is proportional to the surface charge density on the conductor. This is one of the most important assertions in Electromagnetism.

b. Transforming Gauss Law using the linear charge density concept. Cylindrical symmetry

Let's consider a long and very thin bar carrying a uniform linear charge density, λ. We want to find an expression for the electric field E in terms of linear charge density and distance r from the bar. For this, we must consider a cylinder of radius r and height h, and then, find the electric field produced on the lateral surface of it.

Again, the area vector A is parallel to the direction of electric field E. This means cos θ = 1.

From the definition of electric flux, we have

From Gauss Law, we have

Therefore, combining the two above equations, we obtain

Simplifying h from both sides and rearranging in order to isolate E, we obtain

Example 3

What is the electric field produced by a long bar of linear charge density λ = 600 μC/cm at a distance of 4 m from the bar?

Solution 3

Clues:

λ = 600 μC/cm = 60000 μC/m = 0.06 C/m = 6 × 10-2 C/m
r = 4 m
ϵ0 = 8.85 × 10-12 F/m
E = ?

From the equation of electric field in terms of linear charge density, we have

You have reached the end of Physics lesson 15.1.4 Appendix - Charge Density, Electric Field and Gauss Law. There are 4 lessons in this physics tutorial covering Electric Current. Current Density, you can access all the lessons from this tutorial below.

More Electric Current. Current Density Lessons and Learning Resources

Whats next?

Enjoy the "Appendix - Charge Density, Electric Field and Gauss Law" physics lesson? People who liked the "Electric Current. Current Density lesson found the following resources useful:

1. Gauss Law Feedback. Helps other - Leave a rating for this gauss law (see below)
2. Electrodynamics Physics tutorial: Electric Current. Current Density. Read the Electric Current. Current Density physics tutorial and build your physics knowledge of Electrodynamics
3. Electrodynamics Revision Notes: Electric Current. Current Density. Print the notes so you can revise the key points covered in the physics tutorial for Electric Current. Current Density
4. Electrodynamics Practice Questions: Electric Current. Current Density. Test and improve your knowledge of Electric Current. Current Density with example questins and answers
5. Check your calculations for Electrodynamics questions with our excellent Electrodynamics calculators which contain full equations and calculations clearly displayed line by line. See the Electrodynamics Calculators by iCalculator™ below.
6. Continuing learning electrodynamics - read our next physics tutorial: Electric Resistance. Combinations of Resistors

Help others Learning Physics just like you

Please provide a rating, it takes seconds and helps us to keep this resource free for all to use

We hope you found this Physics lesson "Electric Current. Current Density" useful. If you did it would be great if you could spare the time to rate this physics lesson (simply click on the number of stars that match your assessment of this physics learning aide) and/or share on social media, this helps us identify popular tutorials and calculators and expand our free learning resources to support our users around the world have free access to expand their knowledge of physics and other disciplines.

Electrodynamics Calculators by iCalculator™

• Amount Of Substance Obtained Through Electrolysis Calculator
• Charge Density Calculator
• Electric Charge Stored In A Rc Circuit Calculator
• Electric Field In Terms Of Gauss Law Calculator
• Electric Power And Efficiency Calculator
• Electron Drift Velocity Calculator
• Equivalent Resistance Calculator
• Force Produced By An Electric Source Calculator
• Joules Law Calculator
• Ohms Law Calculator
• Potential Difference In Rc Circuit Calculator
• Resistance Due To Temperature Calculator
• Resistance Of A Conducting Wire Calculator